Master's Thesis

I have recalled some basics about measure and integration theory, and this lead me to the idea to take this as the second topic for my master exam. Master exams at the University of Vienna consist of two parts, the first one is about your thesis, and the second one covers a subject which shouldn’t be related to the first part. My first idea had been to take linear algebra with H. Rindler as examiner, who is the dean of the faculty of mathematics. But now I think about deciding for measure and integration theory, the exam of which I had taken at K. Sigmund. The relation to functional analysis is given by measurability, Lebesgue-integrability and the 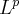-spaces.